

A rational triangle is a Euclidean triangle whose angles are all rational multiples of pi. Rational triangles are parametrized up to scale by the space of triples of integers (p,q,r), where p, q, and r are positive integers with no common factor and listed in increasing order (two may coincide). Any such triangle unfolds to give a translation surface tiled by 2n copies of the triangle, where n = p+q+r. If the Veech group of this surface is a lattice, then the original triangle is called a lattice triangle.
There are several known (and related) infinite families of lattice triangles, but to my knowledge only four known "exceptional" lattice triangles. Three of these have been related to the exceptional Dynkin-Coxeter diagrams E6, E7, and E8 by Chris Leininger, while the fourth was discovered by Pat Hooper and differs from the others by being obtuse. Here we show a sufficiently large portion of the tessellation arising from each of these four exceptional triangles to see a fundamental domain for the action of the Veech group; for each we provide two "rotations", related by a half-turn if n is odd and by a quarter-turn if n is even.




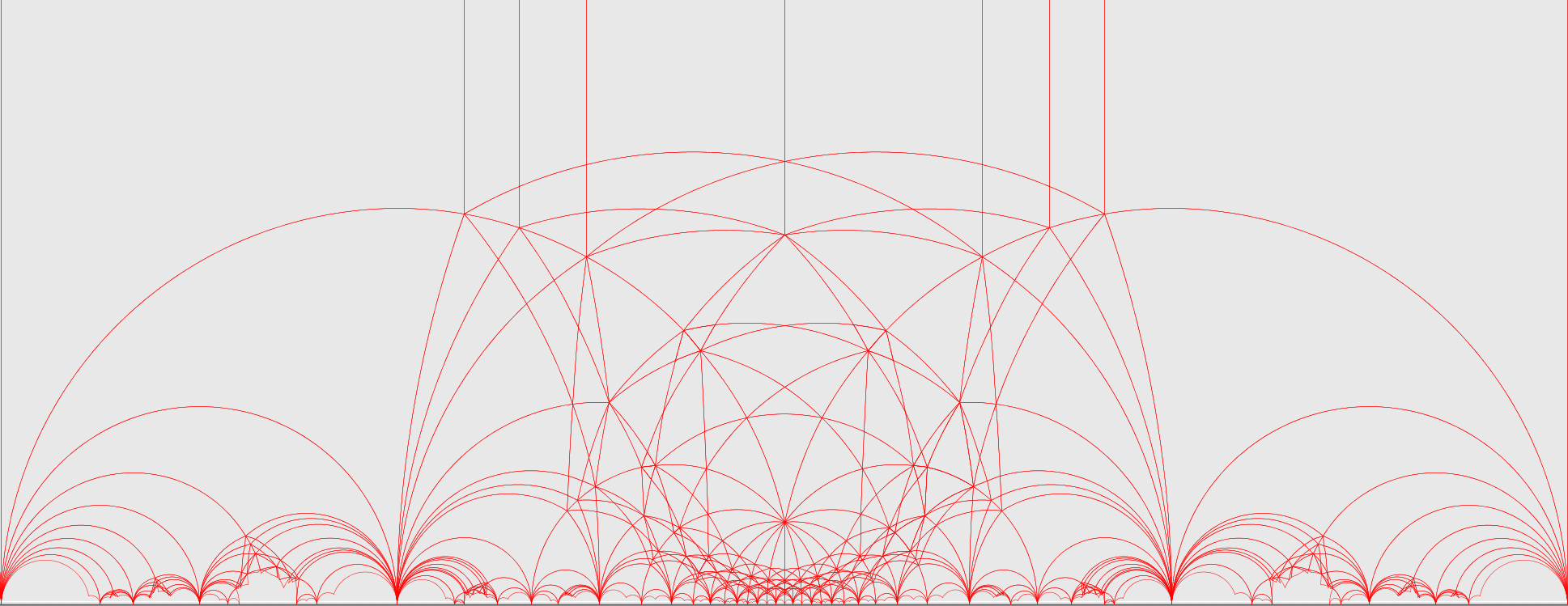
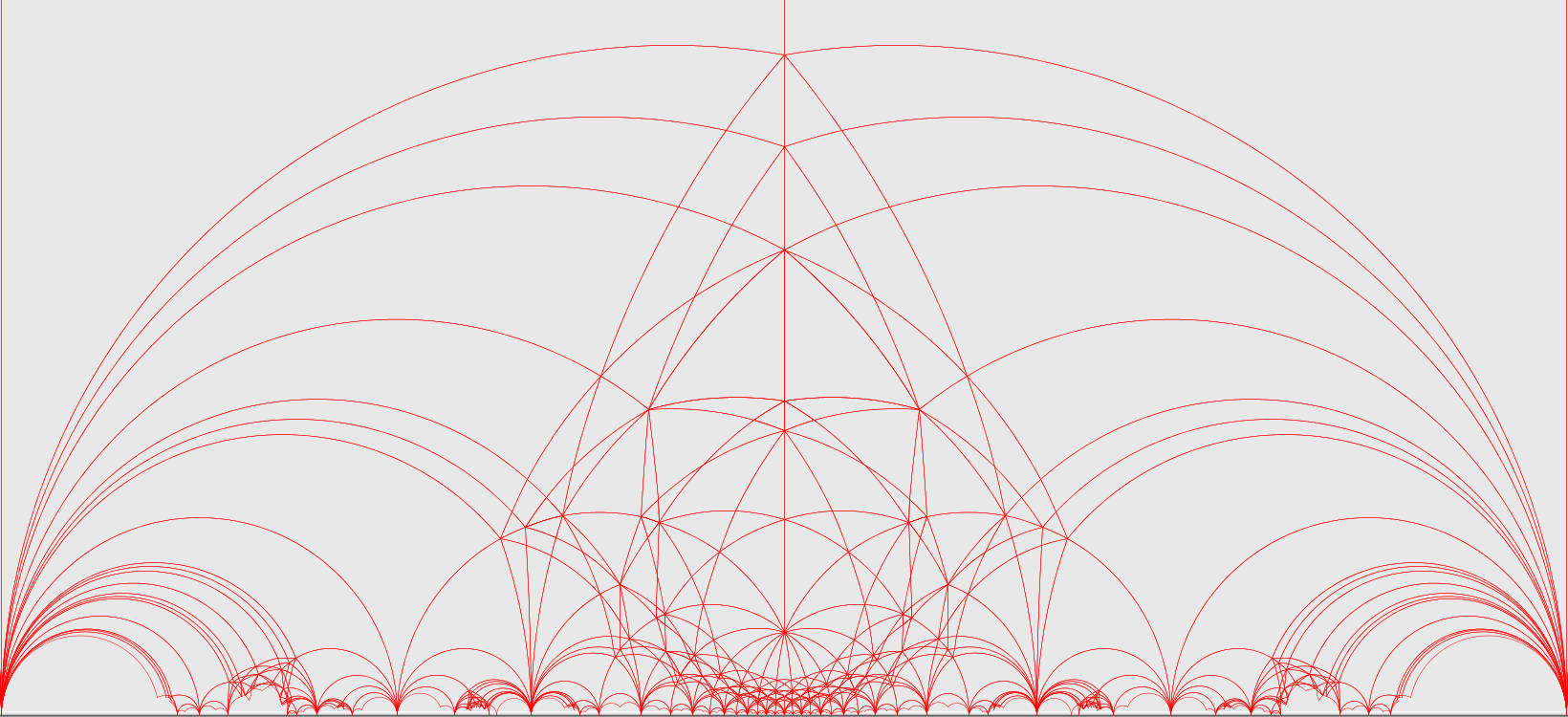


The (1,1,8)-triangle and the (1,2,2)-triangle unfold to give essentially the same surface: it may be thought of as a pair of stellated pentagons glued along their parallel sides, or as a double cover of the regular decagon surface, branched at the central point. It is also commensurable with with Kepler's small stellated dodecahedron (a description is given by Weber), which is naturally a meromorphic quadratic differential on a genus 4 surface.
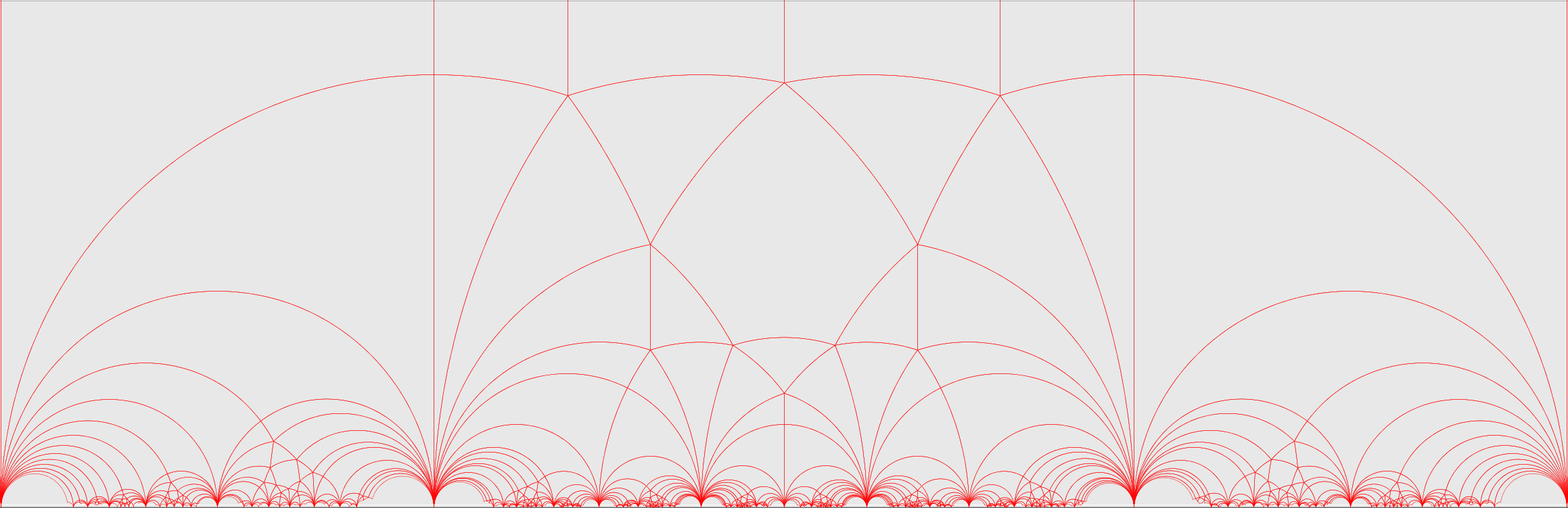
The (1,2,4)-triangle is known not to be a lattice triangle, and in fact its Veech group is unknown; perhaps it only includes the order 7 rotation. Part of my interest in this surface is that it appears as an abelian differential on Klein's quartic curve, the genus 3 surface with the largest possible group of automorphisms.

back to my main page
back to my directory page at the Cornell math department