
Puzzles
---------------------------------------------------------------------------------------------------------------------------------------------------------------------------
13. Find the odd ball
If you have two identical balls, one heavier than the other, you can easily determine which is heavier by putting them on opposite pans of a balance scale. If there are four, all the same weight except for one heavier one, you can find the heavier one in two weighings.
Suppose you have nine identical balls, one of which is heavier than the eight others. What is the smallest number of weighings needed for positively identifying the odd ball?

(Click the link at the bottom of this page for solution)
---------------------------------------------------------------------------------------------------------------------------------------------------------------------------
14. The crazy cut
You are to make one cut (or draw one line)----of course it need not be straight----that will divide the figure into two identical parts.

(Click the link at the bottom of this page for solution)
---------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
15. Racing
Peter and Paul race each other for 100m. Paul wins by 10m. They decide to race again, but this time to even things out, Paul begins 10m behind the start line. Assuming that both run with the same constant speed as before, who wins?
(Click the link at the bottom of this page for solution)
---------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
16. A fixed-point theorem
There are quite a few versions of fixed-point theorems in topology and functional analysis. To understand these, we need to know a lots more advanced mathematics. But this problem is not talking about these theorems. In fact, no advanced mathematics is necessary.
One morning, exactly at sunrise, a Buddhist monk began to climb a tall mountain. The narrow path, no more than a foot or two wide, spiraled around the mountain to a glittering temple at the summit.
The monk ascended the path at varying rates of speed, stopping many times along the way to rest and to eat the dried fruit he carried with him. He reached the temple shortly before sunset. After several days of fasting and meditation, he began his journey back along the same path, starting at sunrise and again walking at variable speeds with many pauses along the way. His average speed descending was, of course, greater than his climbing average speed.
Prove that there is a spot along the path that the monk will occupy on both trips at precisely the same time of day.
(Click the link at the bottom of this page for solution)
-------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
17. Termite and 27 cubes.
Imagine a large cube formed by gluing together 27 smaller wooden cubes of uniform size as shown below. A termite starts at the centre of the face of any one of the outside cubes and bores a path that takes him once through every cube. His movement is always parallel to a side of the large cube, never diagonal.
Is it possible for the termite to bore through each of the 26 outside cubes once and only once, then finish his trip by entering the central cube for the first time? If possible, show how it can be done. If impossible, prove it.
It is assumed that the termite, once it has bored into a small cube, follows a path entirely within the large cube. Otherwise, it could crawl out on the surface of the large cube and move along the surface to a new spot of entry. If this were permitted, there would, of course be no problem.
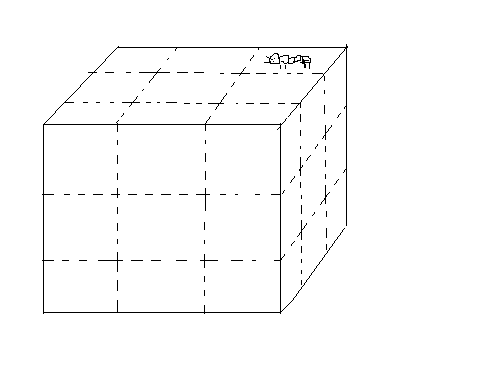
(Click the link at the bottom of this page for solution)
------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
18. The sliced cube and the sliced doughnut
A mathematician, noted for his ability to visualize the three-dimensional structure, was having coffee and doughnuts. Before he dropped a sugar cube into his cup, he placed the cube on the table and thought:
If I pass a horizontal plane through the cube's centre, the cross section will of course be a square. If I pass it vertically through the centre and four corners of the cube, the cross section will be an oblong rectangle. Now suppose I cut the cube this way with the plane......To his surprise, his mental image of the cross section was a rectangular hexagon.
How was the slice made? If the cube's side is half an inch, what is the side of the hexagon?
After dropping the cube into the coffee, the mathematician turned his attention to a doughnut lying flat on a plate.
'If I pass a plane horizontally through the centre, the cross section will be two concentric circles. If I pass the plane vertically through the centre, the section will be two circles separated by the width of the hole. But if I turn the plane so....'
He whistled with astonishment. The section consisted of two perfect circles that intersected!
How was this slice made? If the doughnut is a perfect torus, three inches in outside diameter and with a hole one inch across, what are the diameters of the intersecting circles?
(Click the link at the bottom of this page for solution)
-------------------------------------------------------------------------------------------------------------------------------------------------------------------------------