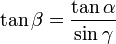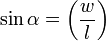Numb3rs 506: Magic Show

This episode is all about mystery and deception. It begins with a magic show including a trick where "The Amazing Talma" disappears from a large glass tube which promptly fills with water in her place. When she fails to reappear, her assistant panics, yells out for someone to call 911 and the magic show immediately ends. But Talma is known for elaborate publicity stunts like this. Was anything really wrong or would she just reappear, unharmed, at a later date?

The FBI originally think that they're being played. However, once they find blood under the stage, it starts to look like a possible murder. The team needs to find out if the blood spatter is the result of a trauma or is just a part of a very well planned magic trick. To do this, Charlie performs an analysis of the spatter pattern using blood spatter trigonometry and the FBI team tests a blood sample to determine its origin.

The results of the tests come back and it turns out that the blood spatter was no hoax; Talma suffered from a sharp force trauma. Soon after this discovery, the FBI team find Talma dead inside of another large glass tube. Her cut is bandaged which indicates that the trauma incurred when disappearing from the magic show was likely an accident. On the other hand, the tube is filled with water, has a broken emergency handle and has a crack in the glass. Was it murder? Charlie and the other two mathematicians reverse engineer the trick in order to try to determine what happened. They deduce that the death was an accident and partly due to the high water pressure needed to fill the tube with water very quickly. All of the signs that originally pointed towards murder were caused by someone trying, but failing, to save Talma. This was one trick and attempt at deception that went horribly wrong.

Blood Spatter Trigonometry

In this episode, the use of blood spatter trigonometry was important in determining whether or not the blood spatter under the stage was from a trauma. In this section, we'll investigate the basics of the field of forensic science called bloodstain pattern analysis.

Brief Overview

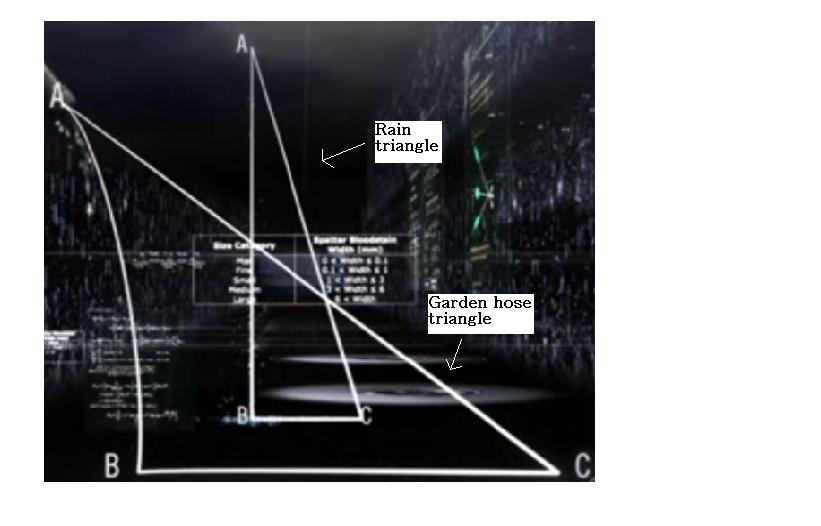
Bloodstain pattern analysis is the analysis of blood spatter patterns at the scene of a crime in order to deduce the events that caused them. Charlie explains the general idea of bloodstain pattern analysis with an example: "It's like when you walk outside and you notice that the ground is wet.", he begins. "The question is, 'Did it rain?' or 'Is the water coming from somewhere else?'. A droplet of water falling in a vertical line from a cloud will slam to the ground and splash outward. Water from a garden hose will have an entirely different mathematical signature: different vectors, velocity, a far more obtuse angle. So we compare the rain triangle to the garden hose triangle to tell us if it rained or if your neighbor just recently washed his car." The following is an image from the episode that accompanies Charlie's exposition.

Through bloodstain pattern analysis, the analyst may be able to determine the movement of the victim during and after bloodshed, the position of the victim during bloodshed, the type of weapon used, and a lower bound on the number of strikes from the weapon.

Bloodstains can be categorized into three main groups: passive stains, projected stains and transfer (or contact) stains. Passive stains are those caused by gravity. Examples include a pool of blood and a drop of blood which has fallen to the ground because of gravity alone. Projected stains are those that are caused by energy being transferred to the blood source. For example, the blood spatter caused by a gunshot is a projected bloodstain. Finally, transfer stains are those that are arise when a bloody object comes into direct contact with a surface without blood. An example of such a stain would be the print left on a wall by a person with a bloody hand.

Projected Bloodstains

Let's take a closer look at projected bloodstains. There are a number of elements which are incorporated into an analysis of such a stain. First, the average size of the stains produced by individual droplets is used to determine how much energy was transferred to the blood source. Small stains correspond to high energy transfer and larger stains to lower energy transfer. For example, a stain with a mist-like appearance could be the result of an explosion or gunshot. Next, analysts use math to determine the angle of impact of a given droplet of blood, the intersection of two or more bloodstain paths and the location of the blood source at the time of trauma. Much of the math that is used to accomplish these tasks is trigonometry. This accounts for the name "blood spatter trigonometry".

Determining the Angle of Impact

When blood is travelling through the air, it has many of the same physical properties as water. For example, a falling droplet of blood has a spherical shape. Thus, if the droplet were to fall to the ground from the vertical, it would form a circular spatter pattern. Similarly, if a drop were to hit any other surface at a 90^o angle, a circular pattern would appear. Of course, in the case of a projected bloodstain, blood can impact a surface at any angle. In order to reconstruct the events of the crime, it is important for the bloodstain pattern analyst to determine this angle. However, because blood is travelling in three dimensional space before it hits a surface (say, a wall), there are multiple angles of interest. These are α, β and γ as labelled in the diagram below. This image comes from the Wikipedia page on bloodstain pattern analysis.

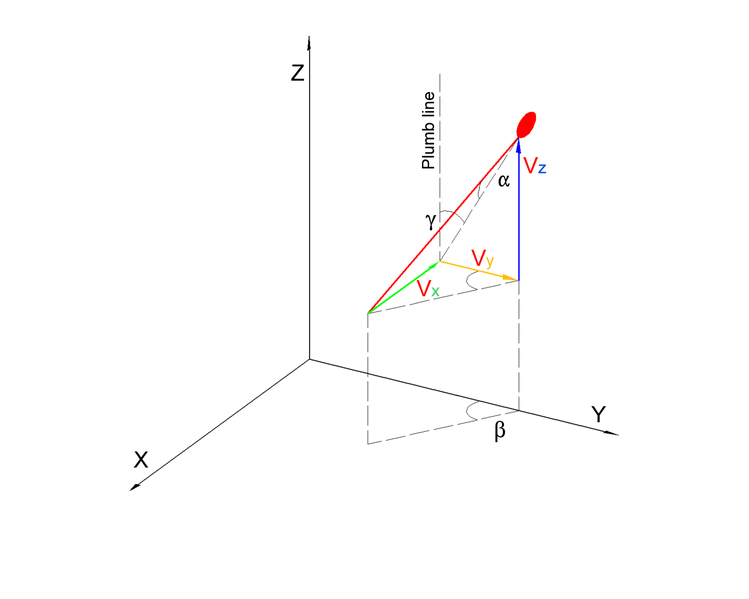
Note that the stained surface corresponds to the yz-plane in the above diagram. Also, the plumb line is the line in the direction of the z-axis which passes through the stain. (For a vertical surface, the plumb line is in the direction of the true vertical.) Finally, α is known as the angle of impact. The three angles satisfy the following relation:

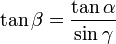
However, how does one go about finding one or more of α, β, γ?

The answer relies on the fact that when a droplet of blood hits a clean surface, it forms a stain in the shape of an ellipse. The line through the major (longer) axis of the ellipse forms an angle with the plumb line. This is the angle γ. To compute α, one needs to measure the length of both the major and minor axes. These are denoted l and w (for length and width) respectively. &alpha is then given by the following formula:

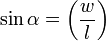
One can then find &beta by using the relation between the three angles.

Finding the Point and Area of Convergence

In a real bloodstain, there are likely many individual spots of blood. And, it is possible that the different droplets of blood that contributed to the stain followed different paths in the air. The intersection (on the stained surface) of two bloodstain paths is known is known as the point of convergence of the paths. Similarly, the area of convergence refers to the area of intersection of multiple bloodstain paths in a given spatter pattern.

To find the point or area of convergence, one draws lines through the major axes of the different elliptical blots of blood and then finds the point or area where the different lines converge. It is important to note that the area of convergence may not be meaningful if the different bloodstain paths were caused by unrelated events.

Locating the Blood Source at the Time of Trauma

The area of origin is the name given to the location of the blood source at the time of trauma. Unlike the two dimensional area of convergence, the area of origin is a region in three dimensional space (despite the name area of origin).

The area of origin can be found as follows: Consider a blood spatter with multiple bloodstain paths. Find the area of convergence of the bloodstain paths. Now, for a single elliptical blot of blood in the spatter pattern, measure the distance from the back-end of the stain to the area of convergence. Denote this distance by D. For this same droplet, compute the angle of impact α. Let H denote the distance from the area of convergence on the surface to the area of origin. H is given by the following formula:

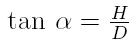
The calculation of H should then be repeated for multiple blots of blood in a given bloodstain. Suppose now that the different values of H which are calculated range from h₁ units to h₂ units. Then, the region in space that is between h₁ and h₂ units from both the area of convergence and the stained surface is the (approximate) area of origin. (Notice that it is not enough to just state that the area of origin is the region in space that is between h₁ and h₂ units from the area of convergence; this would describe a much larger region in space. Why?)

To learn more about bloodstain pattern analysis, check out the following webpages:

Reverse Engineering

Reverse engineering is the process of reconstructing a device (or system) by examining the properties of said device (or system). We won't discuss this topic here. However, the following are a few places where the interested reader can learn more: