 cube |
 tetrahedron |
 octahedron |
 dodecahedron |
 icosahedron |
Introduction
Mathematicians love to classify and count things. We now apply this idea to polyhedra, making some attractive and interesting models along the way.
A regular convex polygon is a closed 2-dimensional figure whose edges have the same length and meet at the same angle at every vertex. For example, a equilateral triangle or a square is a regular convex polygon. There are an infinite number of regular convex polygons.
If we take the same idea and scale it up to three dimensions, we get regular convex polyhedra. That is, a regular convex polyhedron is a closed 3-dimensional object made up of congruent regular convex polygons so that the same number of faces meet at each vertex. However, instead of having an infinite number of regular convex polyhedra, there are only 5, the Platonic solids.
The Platonic solids were known to the Greeks. Plato believed that the cube, tetrahedron, octahedron, and the icosahedron represented the shapes of the fundamental atoms of earth, fire, air, and water. To the fifth regular convex polyhedron, the dodecahedron, he associated the whole universe.
 cube |
 tetrahedron |
 octahedron |
 dodecahedron |
 icosahedron |
Exercise
Use the polydrons to make models of the 5 Platonic solids, and come up with a convincing argument that there are only 5.
Questions
Note: We can relax the requirements slightly and allow the faces to be different regular polygons to get semi-regular convex polyhedra. We insist that the arrangement of the polygons is the same at each vertex. For example, the truncated icosahedron is formed from regular pentagons and hexagons as on a soccer ball. At each vertex we have a pentagon, and then 2 hexagons.
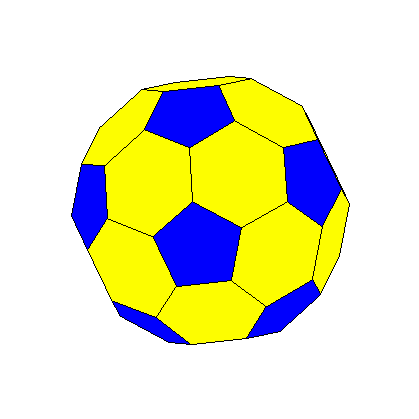
truncated icosahedron
Note: There are 13 very special semi-regular polyhedra called the Archimedean solids. These solids have a lot of symmetry.
Exercises
Perhaps you just realized that the truncated icosahedron is formed by dividing the edges of an icosahedron into thirds and then slicing the corners off of it at these points. We can perform this procedure to each of the Platonic solids and get 4 more Archimedean solids.
Pictures of the polyhedra can be found at http://home.teleport.com/~tpgettys/trplato.shtml
There are 6 more Archimedean solids. One of many sites for reading about and viewing all 13 Archimedean solids is http://home.teleport.com/~tpgettys/archimed.shtml
Now the enquiring mind may want to know whether the prisms, anti-prisms, and Archimedean solids are all of the semi-regular convex polyhedra. There is exactly one more, the pseudorhombicuboctahedron or elongated square gyrobicupola, which is obtained by rotating the bottom third of the small rhombicuboctahedron. Each vertex has a triangle and then three squares. However, it is not considered an Archimedean solid because though each vertex looks the same locally, the polyhedron does not have as many symmetries as the Archimedean solids.
For more information and a picture of the pseudorhombicuboctahedron visit http://euch3i.chem.emory.edu/proposal/www.li.net/~george/virtual-polyhedra/pseudo-rhombicuboctahedra.html
There is a lot of information on-line about polyhedra and much of it is even correct. With the right software, you can even view virtual 3-dimensional models and rotate them with your mouse. You might want to start with the following sites:
To build your own paper models of the Platonic solid earth maps, print out the patterns on card stock from http://ccins.camosun.bc.ca/~jbritton/polymap/jbpolymap.htm