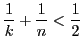|
9. Tesselations of the Euclidean and non-Euclidean plane
Definition 1
A plane tessellation is an infinite set of polygons fitting together to cover the whole plane just once, so that every side of the polygon belogs also to another polygon. No two of the polygons have common interior points.
We will analyse only regular tessellations.
Definition 2
A tessellation is called regular if its faces are regular and equal. The same number of polygons meet at each vertex. We denote with
(There are also quasiregular tessellation - built from two kinds of regular polygons so that two of each meet at each vertex, alternately.)
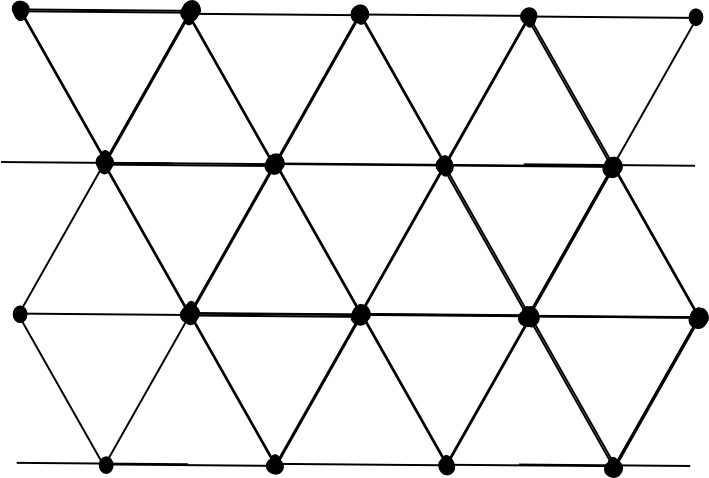
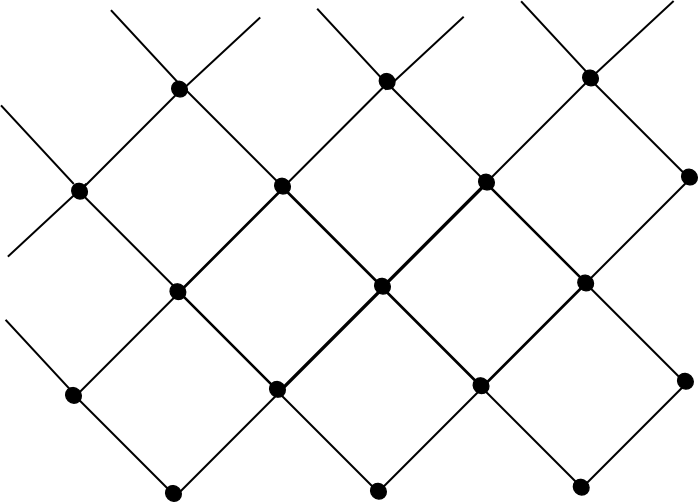
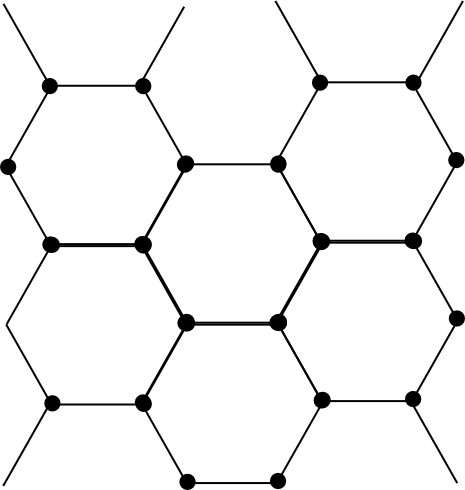
Regular tessellations of the Euclidean plane
This equation has only 3 solutions:
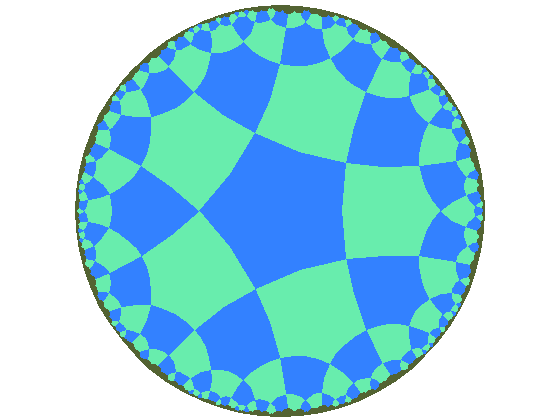
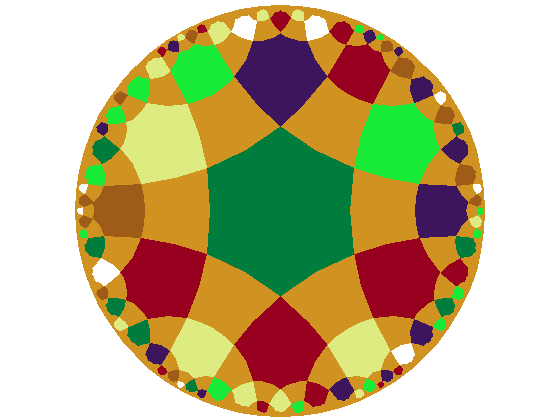
Regular tessellations of the hyperbolic plane
But this happens for infinitely many pairs of
Remember that even if it may look like the sides of the pentagons are curved, they are not - the effect is due to the representation we're using. In the actual hyperbolic plane they would be straight. Also, the pentagon in the middle looks larger, but in fact it isn't. All the pentagons are absolutely identical (in the hyperbolic world).
We started with Escher, let's finish with Escher. Here are two "regular divisions of the plane" (that's the name Escher used for tessellations):
|
 where
where