The region examined here is the Euclidean Regular Six-Star of side length 1 with Dirichlet Boundary conditions. To clarify what is meant by Six-Star, we define it to be a twelve-sided polygon in R^2 with alternating concave and convex angles. A regular six-star is composed of twelve equilateral triangles where six triangles form a regular hexagon and the other six triangles share sides with the regular hexagon. A Star of David is a regular Six-Star. All Eigenvalues of the Euclidean Equilateral Triangle under Dirichlet Boundary conditions are also eigenvalue of this region, which is why we have divided the values in the table below by a factor of (4/3*pi)^2, so that we can observe the common values. This has a dual purpose of easily allowing us to check the accuracy of the Six-Star eigenvalues: since 13 = 3^2 + 1^2 + 3*1 is an eigenvalue of multiplicity two in the Equilateral Triangle, it should also be an eigenvalue of multiplicity two in the six-star, and indeed we see that our predictions for eigenvalues 85 and 86 differ from 13 by less than .0004. This was an extremely important test case for us: it allowed us to extend our theory to non-convex geometric regions of R^2 by giving us some known eigenvalues to test if our estimations were accurate, namely those shared with the Euclidean Equilateral Triangle.
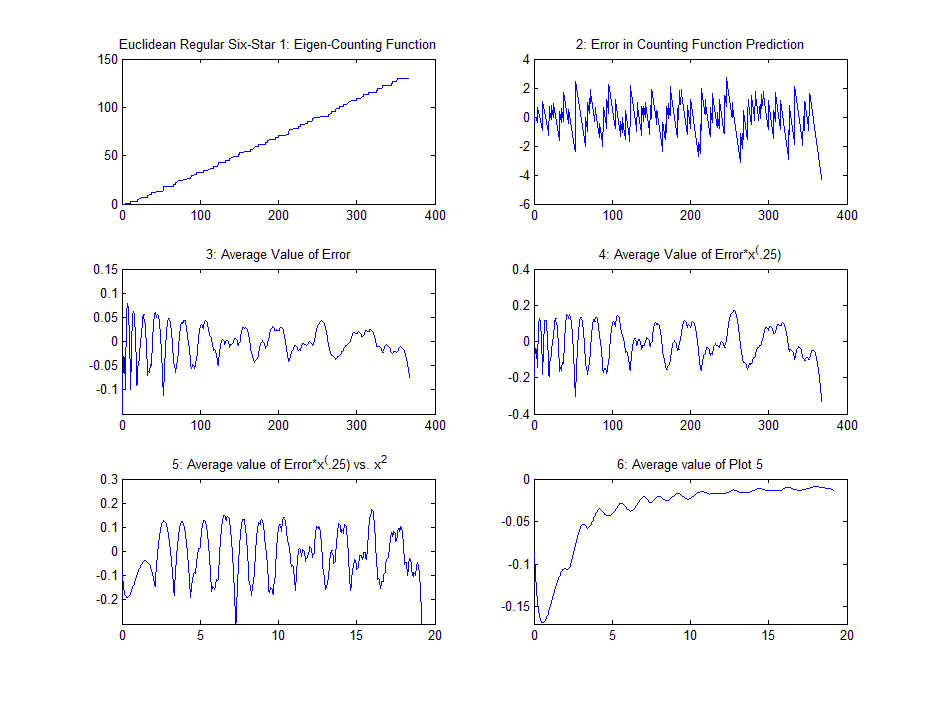
Here are the counting graphs for the eigenvalue
spectrum, using the predicted values. The first approximately 130 eigenvalues
values were used here. The equation for the predicted counting function is N'(t) = 3sqrt(3)/4pi*t - 3/pi*sqrt(t) + 25/48.

Here are the difference graphs for the eigenvalue
spectrum, using the predicted values. The first approximately 130 values were used here.

Below is the Eigenvalue spectrum for the Euclidean Regular Six-Star of side length 1
under Dirichlet Boundary conditions. We have removed a factor of (4/3*pi)^2 from the figures in order to easily compare the
values to those of the Euclidean Equilateral Triangle. The calculated and predicted eigenvalue spectrum is presented here.
| Euclidean Six-Star under Dirichlet Boundary Conditions | ||||||||
|---|---|---|---|---|---|---|---|---|
| Refinement | ||||||||
| # | Initial Value | 1 | 2 | 3 | 4 | 5 | Predicted Value | |
| 1 | 0.274817489 | 0.260728357 | 0.256563678 | 0.255256962 | 0.254833377 | 0.254692963 | 0.2546452 | |
| 2 | 0.710560585 | 0.655202154 | 0.64030984 | 0.636040824 | 0.634767653 | 0.634375597 | 0.63424223 | |
| 3 | 0.711187282 | 0.655340476 | 0.640350875 | 0.636053989 | 0.634772042 | 0.634377136 | 0.63424279 | |
| 4 | 1.266083459 | 1.138649419 | 1.105292003 | 1.096313926 | 1.093829822 | 1.093124019 | 1.0928839 | |
| 5 | 1.268034848 | 1.13905236 | 1.1053963 | 1.096342195 | 1.093837801 | 1.093126355 | 1.0928843 | |
| 6 | 1.506567148 | 1.321596968 | 1.276613516 | 1.26484021 | 1.261639302 | 1.260742914 | 1.260438 | |
| 7 | 1.636634158 | 1.477506845 | 1.433930384 | 1.422802924 | 1.419991394 | 1.419284622 | 1.4190442 | |
| 8 | 2.327051819 | 1.976703658 | 1.893022759 | 1.872226465 | 1.866953743 | 1.865602378 | 1.8651427 | |
| 9 | 2.330486683 | 1.977719504 | 1.893273814 | 1.872289158 | 1.86696953 | 1.865606367 | 1.8651426 | |
| 10 | 2.707883402 | 2.173059249 | 2.048867322 | 2.016387715 | 2.007398525 | 2.004817589 | 2.0039396 | |
| 11 | 2.757646756 | 2.305205769 | 2.192647401 | 2.164401873 | 2.157162772 | 2.155281997 | 2.1546422 | |
| 12 | 2.758807137 | 2.306151741 | 2.192920683 | 2.164471006 | 2.157179414 | 2.155285873 | 2.1546417 | |
| 13 | 3.382381162 | 2.726701975 | 2.573958182 | 2.536837735 | 2.527612992 | 2.525306023 | 2.5245212 | |
| 14 | 4.122792886 | 3.286512844 | 3.070281228 | 3.017478599 | 3.004364187 | 3.001090727 | 2.9999771 | |
| 15 | 4.252680083 | 3.328458161 | 3.097178241 | 3.039298946 | 3.024286091 | 3.020309849 | 3.0189572 | |
| 16 | 4.292922843 | 3.333185003 | 3.098014445 | 3.03947665 | 3.024329463 | 3.020321247 | 3.0189577 | |
| 17 | 4.393185392 | 3.359523312 | 3.110786499 | 3.049056974 | 3.032994476 | 3.028693999 | 3.027231 | |
| 18 | 4.403599582 | 3.362471511 | 3.111680551 | 3.04931823 | 3.0330668 | 3.028713833 | 3.027233 | |
| 19 | 5.324392396 | 4.205782857 | 3.871184074 | 3.788353456 | 3.767473268 | 3.762157801 | 3.7603496 | |
| 20 | 5.341756048 | 4.211122433 | 3.872330606 | 3.788626111 | 3.767540178 | 3.762174329 | 3.7603489 | |
| 21 | 5.661281678 | 4.397844127 | 4.030977922 | 3.941359588 | 3.918908555 | 3.913217275 | 3.9112812 | |
| 22 | 5.683083729 | 4.401219376 | 4.031604904 | 3.941500133 | 3.918942751 | 3.913225824 | 3.911281 | |
| 23 | 6.870620746 | 4.583529172 | 4.1731211 | 4.069946771 | 4.043578199 | 4.036683394 | 4.0343379 | |
| 24 | 7.036812817 | 4.597179434 | 4.211620097 | 4.118220837 | 4.094858655 | 4.089004317 | 4.0870128 | |
| 25 | 7.602909405 | 5.115790114 | 4.608495484 | 4.485548858 | 4.454332789 | 4.446222434 | 4.4434634 | |
| 26 | 7.639876882 | 5.480923556 | 4.950076899 | 4.820479513 | 4.788357138 | 4.78034162 | 4.7776149 | |
| 27 | 7.867040242 | 5.832767787 | 5.223780765 | 5.077177472 | 5.04083521 | 5.031717899 | 5.0286163 | |
| 28 | 7.92101847 | 5.839702146 | 5.225464343 | 5.077604408 | 5.040942813 | 5.0317448 | 5.0286158 | |
| 29 | 7.939654095 | 5.987604541 | 5.304215277 | 5.13781803 | 5.095354759 | 5.084226387 | 5.0804407 | |
| 30 | 8.862892087 | 6.179590579 | 5.520619809 | 5.360866027 | 5.32130633 | 5.311427078 | 5.3080663 | |
| 31 | 8.925261988 | 6.185230623 | 5.521911844 | 5.361193225 | 5.321388628 | 5.311447652 | 5.3080659 | |
| 32 | 9.111565238 | 6.456918754 | 5.699004428 | 5.514970817 | 5.468355024 | 5.456277431 | 5.4521688 | |
| 33 | 9.177824353 | 6.471679984 | 5.702246029 | 5.515755841 | 5.468550739 | 5.456326616 | 5.4521681 | |
| 34 | 10.55854592 | 7.038772242 | 6.194985573 | 5.992920293 | 5.943140182 | 5.93073733 | 5.9265181 | |
| 35 | 11.06507097 | 7.057060209 | 6.198603499 | 5.993769491 | 5.943349347 | 5.930789193 | 5.9265164 | |
| 36 | 17.04972554 | 7.638125482 | 6.636002109 | 6.397901761 | 6.338887047 | 6.323994733 | 6.3189286 | |
| 37 | 0 | 7.813884996 | 6.762390154 | 6.515013028 | 6.453793819 | 6.438338982 | 6.4330815 | |
| 38 | 0 | 8.133372726 | 7.024212768 | 6.756365406 | 6.689661175 | 6.672723946 | 6.6669621 | |
| 39 | 0 | 8.16135409 | 7.029624839 | 6.757594179 | 6.689959249 | 6.672797467 | 6.6669593 | |
| 40 | 0 | 8.531347454 | 7.370223407 | 7.091390043 | 7.0227754 | 7.005689419 | 6.999877 | |
| 41 | 0 | 8.581845678 | 7.381896177 | 7.094094938 | 7.023433102 | 7.00585242 | 6.9998717 | |
| 42 | 0 | 8.751645987 | 7.436179888 | 7.12035055 | 7.040407946 | 7.019537619 | 7.0124378 | |
| 43 | 0 | 8.761957191 | 7.438848308 | 7.121060115 | 7.040603433 | 7.019592332 | 7.0124447 | |
| 44 | 0 | 9.328156936 | 7.945058757 | 7.615667895 | 7.534720502 | 7.514511865 | 7.5076372 | |
| 45 | 0 | 9.336949272 | 7.946742335 | 7.61612669 | 7.534840187 | 7.514542641 | 7.5076377 | |
| 46 | 0 | 9.768677062 | 8.31780774 | 7.963891579 | 7.876767836 | 7.855065408 | 7.8476826 | |
| 47 | 0 | 9.794172385 | 8.322852205 | 7.965055949 | 7.877052802 | 7.85513608 | 7.8476803 | |
| 48 | 0 | 9.968299615 | 8.495581392 | 8.126539245 | 8.035158683 | 8.01233577 | 8.0045717 | |
| 49 | 0 | 10.24718256 | 8.641349393 | 8.249119006 | 8.151994103 | 8.127483622 | 8.1191455 | |
| 50 | 0 | 10.70668369 | 9.001578117 | 8.5889191 | 8.487657633 | 8.462451835 | 8.4538772 | |
| 51 | 0 | 10.71331656 | 9.002234108 | 8.589114017 | 8.487707787 | 8.462464374 | 8.4538769 | |
| 52 | 0 | 10.97622717 | 9.161796735 | 8.726295997 | 8.61877155 | 8.591657052 | 8.5824331 | |
| 53 | 0 | 10.99856963 | 9.166787626 | 8.727487154 | 8.619063355 | 8.591728863 | 8.5824301 | |
| 54 | 0 | 11.7637878 | 9.636834202 | 9.128583397 | 9.001020724 | 8.967918523 | 8.9566576 | |
| 55 | 0 | 12.14621707 | 10.07047582 | 9.559213499 | 9.433834804 | 9.402613948 | 9.3919931 | |
| 56 | 0 | 12.17781694 | 10.07788265 | 9.560966039 | 9.434267382 | 9.402721665 | 9.3919903 | |
| 57 | 0 | 12.37776834 | 10.26906509 | 9.740109808 | 9.609238681 | 9.57638611 | 9.5652101 | |
| 58 | 0 | 12.61129784 | 10.3588373 | 9.820270125 | 9.688528143 | 9.65577531 | 9.6446333 | |
| 59 | 0 | 12.69896472 | 10.40252029 | 9.853774698 | 9.720056762 | 9.686836586 | 9.6755356 | |
| 60 | 0 | 12.93010336 | 10.57149191 | 9.990805646 | 9.847949426 | 9.811984459 | 9.7997497 | |
| 61 | 0 | 13.03526715 | 10.69485305 | 10.11475039 | 9.971732313 | 9.935788433 | 9.9235608 | |
| 62 | 0 | 13.05063193 | 10.7048468 | 10.11738177 | 9.972398563 | 9.935955423 | 9.923558 | |
| 63 | 0 | 13.88084853 | 11.29633271 | 10.66029126 | 10.50453577 | 10.46574053 | 10.452543 | |
| 64 | 0 | 13.89196619 | 11.29954598 | 10.66109828 | 10.5047381 | 10.46579125 | 10.452542 | |
| 65 | 0 | 14.21912349 | 11.44664876 | 10.78120625 | 10.61843491 | 10.57765459 | 10.563782 | |
| 66 | 0 | 14.2460767 | 11.44896952 | 10.78170836 | 10.61856656 | 10.57768992 | 10.563784 | |
| 67 | 0 | 14.40129131 | 11.61524538 | 10.93189903 | 10.7653023 | 10.72382724 | 10.709718 | |
| 68 | 0 | 15.29281375 | 12.17359374 | 11.41811228 | 11.23356955 | 11.18733499 | 11.171607 | |
| 69 | 0 | 15.3101106 | 12.17426683 | 11.41826788 | 11.23361572 | 11.18734867 | 11.171609 | |
| 70 | 0 | 15.61446038 | 12.43859031 | 11.66398935 | 11.47469054 | 11.42728476 | 11.411158 | |
| 71 | 0 | 15.69342156 | 12.45528531 | 11.6677982 | 11.47561839 | 11.42751502 | 11.411151 | |
| 72 | 0 | 16.21316276 | 13.09670977 | 12.26973551 | 12.0670946 | 12.01675197 | 11.999626 | |
| 73 | 0 | 16.6488567 | 13.26734788 | 12.40290631 | 12.18939453 | 12.13573888 | 12.117486 | |
| 74 | 0 | 16.709119 | 13.28031382 | 12.40483724 | 12.18979291 | 12.13583406 | 12.117478 | |
| 75 | 0 | 16.7531257 | 13.35021708 | 12.47030814 | 12.25561774 | 12.20187318 | 12.18359 | |
| 76 | 0 | 16.7580898 | 13.36314826 | 12.47434725 | 12.25666869 | 12.20213877 | 12.183588 | |
| 77 | 0 | 17.29813311 | 13.41766051 | 12.49834707 | 12.27376379 | 12.21705901 | 12.197769 | |
| 78 | 0 | 17.54342485 | 13.68950538 | 12.76067749 | 12.53607883 | 12.48034464 | 12.461385 | |
| 79 | 0 | 17.77206604 | 14.13707556 | 13.16271868 | 12.92363463 | 12.86374222 | 12.843368 | |
| 80 | 0 | 17.79629954 | 14.14364516 | 13.16450599 | 12.92409286 | 12.86385849 | 12.843368 | |
| 81 | 0 | 18.14090758 | 14.30357824 | 13.31880758 | 13.07919406 | 13.01976615 | 12.99955 | |
| 82 | 0 | 18.21781985 | 14.31518775 | 13.32098814 | 13.07971441 | 13.01989552 | 12.999546 | |
| 83 | 0 | 18.41820782 | 14.70436558 | 13.67622084 | 13.42348179 | 13.36056818 | 13.339166 | |
| 84 | 0 | 19.04926123 | 14.93212794 | 13.85099266 | 13.58802049 | 13.52257296 | 13.500309 | |
| 85 | 0 | 19.09755097 | 14.94509046 | 13.85430282 | 13.58885259 | 13.52278099 | 13.500304 | |
| 86 | 0 | 19.62406695 | 15.36306067 | 14.2220176 | 13.94424488 | 13.8750717 | 13.85154 | |
| 87 | 0 | 19.81925657 | 15.42689701 | 14.2814136 | 14.00164156 | 13.93180726 | 13.908051 | |
| 88 | 0 | 19.84918368 | 15.43372251 | 14.2824617 | 14.00187466 | 13.93186425 | 13.908048 | |
| 89 | 0 | 20.20941925 | 15.55258746 | 14.39491777 | 14.11565239 | 14.04649119 | 14.022964 | |
| 90 | 0 | 20.23430303 | 15.55714007 | 14.39620353 | 14.11596985 | 14.04656984 | 14.022961 | |
| 91 | 0 | 0 | 16.14907508 | 14.85088361 | 14.53517453 | 14.4558332 | 14.428842 | |
| 92 | 0 | 0 | 16.83871689 | 15.48249669 | 15.15385187 | 15.07207922 | 15.044261 | |
| 93 | 0 | 0 | 16.84970176 | 15.4851446 | 15.15449646 | 15.07223766 | 15.044254 | |
| 94 | 0 | 0 | 16.96763886 | 15.62842199 | 15.30132967 | 15.22016 | 15.192547 | |
| 95 | 0 | 0 | 17.07301523 | 15.69609397 | 15.36335989 | 15.28064343 | 15.252504 | |
| 96 | 0 | 0 | 17.07647585 | 15.69723098 | 15.36364599 | 15.28071638 | 15.252505 | |
| 97 | 0 | 0 | 17.4290635 | 16.00275653 | 15.65797922 | 15.57266045 | 15.543636 | |
| 98 | 0 | 0 | 17.44258114 | 16.00598462 | 15.65876744 | 15.57285594 | 15.54363 | |
| 99 | 0 | 0 | 17.61771715 | 16.08903278 | 15.71469825 | 15.61978639 | 15.587499 | |
| 100 | 0 | 0 | 17.81055695 | 16.32341034 | 15.96327964 | 15.87407508 | 15.843729 | |
| 101 | 0 | 0 | 17.82167575 | 16.3264646 | 15.96406159 | 15.8742717 | 15.843726 | |
| 102 | 0 | 0 | 18.19262089 | 16.61055387 | 16.22666159 | 16.13091592 | 16.098345 | |
| 103 | 0 | 0 | 18.19472394 | 16.61162877 | 16.22695396 | 16.13098944 | 16.098344 | |
| 104 | 0 | 0 | 18.53386747 | 16.91109594 | 16.52074803 | 16.42417368 | 16.39132 | |
| 105 | 0 | 0 | 18.65065901 | 17.04154303 | 16.65098369 | 16.55426628 | 16.521364 | |
| 106 | 0 | 0 | 18.7191779 | 17.08733932 | 16.69744623 | 16.60110897 | 16.568336 | |
| 107 | 0 | 0 | 18.8658122 | 17.2102884 | 16.81294914 | 16.71477671 | 16.68138 | |
| 108 | 0 | 0 | 19.36251847 | 17.63380176 | 17.21624077 | 17.11297143 | 17.077841 | |
| 109 | 0 | 0 | 19.38143678 | 17.63867012 | 17.21745358 | 17.11327406 | 17.077834 | |
| 110 | 0 | 0 | 19.91811578 | 18.06220286 | 17.60855664 | 17.49533972 | 17.456825 | |
| 111 | 0 | 0 | 19.94775735 | 18.0662471 | 17.62046992 | 17.51039161 | 17.472945 | |
| 112 | 0 | 0 | 20.07423659 | 18.18584726 | 17.73016809 | 17.61662858 | 17.578004 | |
| 113 | 0 | 0 | 20.09690904 | 18.19054121 | 17.73130111 | 17.61691127 | 17.577998 | |
| 114 | 0 | 0 | 20.50874792 | 18.57455319 | 18.11130761 | 17.99686932 | 17.957939 | |
| 115 | 0 | 0 | 20.51523659 | 18.57603558 | 18.11166894 | 17.99695823 | 17.957935 | |
| 116 | 0 | 0 | 0 | 19.22716027 | 18.72465324 | 18.59907108 | 18.55635 | |
| 117 | 0 | 0 | 0 | 19.23578334 | 18.72649469 | 18.59951277 | 18.556315 | |
| 118 | 0 | 0 | 0 | 19.2957732 | 18.7760833 | 18.64630359 | 18.602154 | |
| 119 | 0 | 0 | 0 | 19.30041017 | 18.77721575 | 18.64658172 | 18.602142 | |
| 120 | 0 | 0 | 0 | 19.67914058 | 19.16406143 | 19.0357037 | 18.992038 | |
| 121 | 0 | 0 | 0 | 19.68397759 | 19.16428142 | 19.03578805 | 18.992076 | |
| 122 | 0 | 0 | 0 | 19.68683352 | 19.1688574 | 19.0420875 | 18.998962 | |
| 123 | 0 | 0 | 0 | 19.69274143 | 19.17132065 | 19.04271044 | 18.998959 | |
| 124 | 0 | 0 | 0 | 20.32298953 | 19.77531769 | 19.63967281 | 19.593528 | |
| 125 | 0 | 0 | 0 | 0 | 19.778735 | 19.64051631 | 19.593496 | |
| 126 | 0 | 0 | 0 | 0 | 19.92212753 | 19.78366776 | 19.736566 | |
| 127 | 0 | 0 | 0 | 0 | 19.92345604 | 19.7840006 | 19.73656 | |
| 128 | 0 | 0 | 0 | 0 | 20.22431156 | 20.08123763 | 20.032566 | |
| 129 | 0 | 0 | 0 | 0 | 20.22590794 | 20.08163259 | 20.032552 | |
| 130 | 0 | 0 | 0 | 0 | 20.29934477 | 20.1443245 | 20.091589 | |
| 131 | 0 | 0 | 0 | 0 | 0 | 20.14708981 | ||
| 132 | 0 | 0 | 0 | 0 | 0 | 20.49548504 |