Triangular Billiards
Consider an equilateral triangular billiard table and assume that a billiard ball starts from
one corner of the triangle, say A. The ball bounces 7 times obeying the same rules of billiards
and returns to the original corner. Consider also that the initial triangle has vertices located
at A=(0,0), B=(1,0), and C=(0,1), as in the picture.
Question: Find an angle for which the ball returns to the initial vertex A?
This is one of the problems proposed by Ken Duisenberg regarding the path of a billiard ball
in an equilateral triangle, Reference #9.
In order to answer the question, we need to tesselate one sixth of the plane with
unfoldings of the billiard table, as shown in the image below. Each vertex of the tesselation
corresponds to one of the original veritices of the equilateral billiard table. For
example, point of coordinates (0,0), (1,1), (0,3), (1,4), (2,2) all correspond to vertex A.
Points of coordinates (1,0), (0,2), (2,1) correspond to vertex B, and similarly for C, as
shown in the picture below.
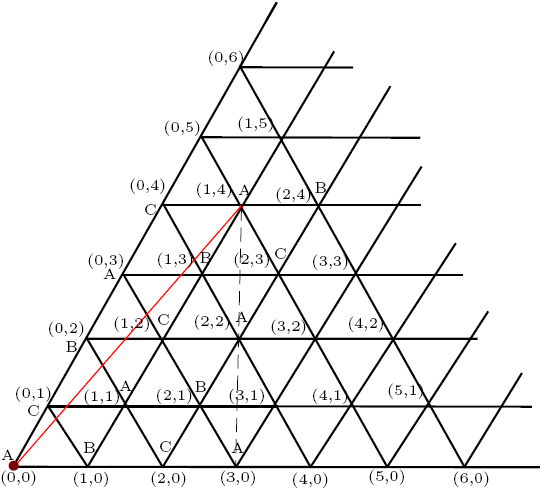
Remark #1: A line drawn from the origin to vertex A of
coordinates (1,4) represents a ball that returns to corner A after a number of bounces.
Remark #2: The number of tesselation edges that the red line
in the picture crosses represents the number of bounces. In this case the red line illustrates
exactly 7 bounces.
The problem now reduces to finding an angle in the right triangle with vertices at
(0,0), (1,4), and (0,3). Notice however that the distance from (1,4) to (0,3)
is exactly four times the height in an equilateral triangle of sidelength 1. It is
therefore 2√3. The distance between (0,0) and (0,3) is just 3 and thereofre the tangent
of the angle that we want is 2√3 ⁄ 3. The angle is then arctan(2√3 ⁄ 3), which is
approximately 49.11 degrees.
Exercise #1: Find another angle which also solves the problem.
Exercise #2: Is it possible to solve the problem if we ask for 5 bounces instead of 7?
As a hint, the answer to this question is negative.
Exercise #3: For what initial angles will the ball starting from A return to A? How many
bounces are necessary for this to happen?