Teaching

Math 1120 Calculus II, Fall 2011

Welcome to the Section 7 Website

The activities on 11/22 were selected from the notes and exercises available at Jim Belk's Calculus 2 Webpage
Specifically, exercises were taken from:
Computing Taylor Series
Convergence of Power Series
Applications of Taylor Series.
Solutions to Practice Problems.
I encourage you to explore these notes and the website further, particularly if you do not feel confident with recent material.

The review activity distributed on 11/22 is from the same website, Practice Exam 2. Note that I do not recommend problems 6 and 7 for this class.

Class Notes on Series
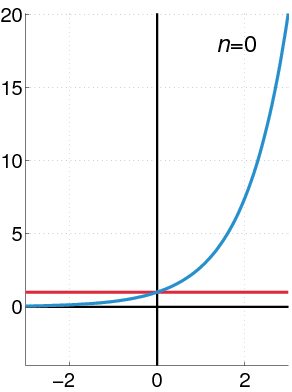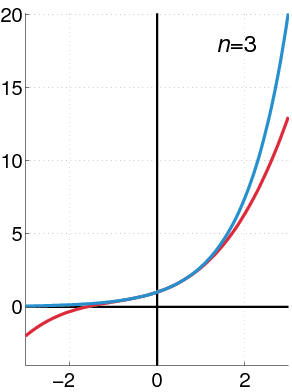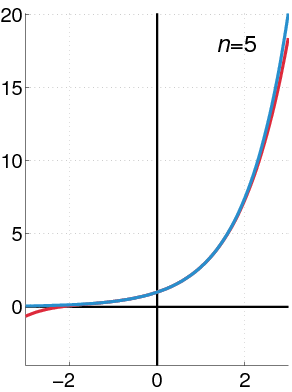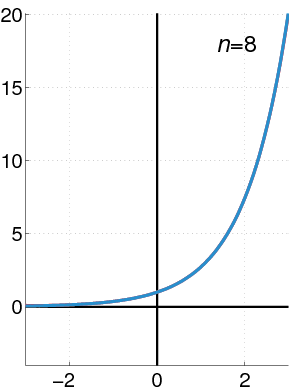
These notes review improper integrals and the various tests we have for series convergence. They then continue into our study of power series and Taylor series from 11/15. The notes include the computation of several Taylor series as well as images of them converging within their radius of convergence.

Fill-in-the-blank Notes on Power Series
What are power series? When do they converge, and to what? What is the mystery function? See if you can answer these questions on your own. Then check the solutions. *Note: The solutions imply that a convergent taylor series always converges to the function f. Can you recall an example for which this is not the case?

A Friend In Need....
Help your friend figure out why she lost points on a recent test on series. In so doing, learn about the common mistakes that students make on series problems. Here is a summary of the corrections necessary.

Collection of Series from Past Prelims
Distributed in class on 11/9, you are expected to finish this activity on your own. It's important that you practice determining the convergence of series, as this skill will be featured heavily on the final! Here are the solutions.

Solutions to Introductory Collection of Series
These are the answers to the series we worked on n class on 11/1, along with an solution to the first quiz question on series.

Sigma Notation and Series
This weekend activity is designed to introduce you to series. If you missed us discussing the answers in class, here are some solutions.
To Converge or Not to Converge
This corresponding activity relates the examples in the activity above to the tests we've been learning about in class.

Note on L'Hospital's Rule
Feeling uncomfortable with limits and L'Hospital's? Now is the time to become an expert! This note explains the notion we've discussed in class of functions fighting over the limit.
Limits at Inifinity Notes
Courtesy of Jim Belk (Bard College), the notes are designed to develop your intuition for limits. Be warned, however, to not use these intuitive ideas as justification on exams: you should always refer to a known limit or use L'Hospital's rule when writing up solutions.

Numerical Integration Problems
Two problems that we did together on the overhead in class, and a guide to deriving Simpson's Rule.

Get Your Integral On
These integrals have been selected from past prelims at Cornell to practice your integration techniques. They are NOT ordered by integration technique, so you can practice the essential skill of figuring out the right technique on your own. There are some partial solutions here, but don't peek until you've tried them all!

A Difficult Partial Fractions Example
This is a challenging problem that needed correction after class. Included is a guide on how to finish up any partial fractions problem.

Arc Length and Volumes Activity
A few problems on arc length discussed in class; here are solutions.

Volumes Activity
A challenging activity in which we computed the volume of the great pyramid using the method of slicing. It was then possible to acertain the average number of blocks placed each day during the two decades of construction. A few other problems are included, such as the volume of a fishbowl. Here are some solutions .

I-clicker Questions from Class
These questions are from the Good Questions Project. If you've decided on your final answer to each question, you can check out the solutions. Try coming up with a counterexample for every false statement.

U- substitution
This is the classroom activity from 8/30 to practice our first integration technique. Here are the Solutions

Review of Calculus I
Ask your instructor about any questions that you feel unsure about.

Back to the Math 1120 Website .