Lionel Levine

Professor
Department of Mathematics
Cornell University

CV | Research | Papers | Talks | Teaching | Gallery | Fun! | Contact

New Course: Math for AI Safety (Fall 2024)

Hello, I'm Lionel! I study abelian networks. These are interacting particle systems whose final state does not depend on the order of interactions. From another point of view, they are systems of communicating automata that pass messages to perform an asynchronous computation. I was inspired to work in this area by Deepak Dhar, Cris Moore, Yuval Peres, and Jim Propp.

If you want to learn a bit about this fascinating nexus of math, computer science, and statistical physics, I recommend starting with WHAT IS a sandpile? for a non-technical overview, and Laplacian growth, sandpiles, and scaling limits for a more recent survey.

Some highlights of my research are the scaling limit of the abelian sandpile in Z^2 where an Apollonian circle packing makes a surprise appearance, the devil's staircase for parallel chip-firing, refuting the density conjecture for sandpiles, logarithmic fluctuations for internal DLA, asynchronous circuits with integer input and output, fast simulation of growth models, a generalization of Knuth's formula for spanning trees, and word equations in uniquely divisible groups.

I thank Open Philanthropy, National Science Foundation, Sloan Foundation, Simons Foundation, and Institute for Advanced Study for supporting my research.

I'm an editor of Combinatorial Theory, a open-source journal with no author fees and double-blind refereeing. Please submit your work to CT!

Teaching:

(upcoming) MATH 6720: Graduate Probability II, Spring 2025
(current) MATH 7710: Topics in Probability: Math for AI Safety, Fall 2024
MATH 1340: Strategy, Cooperation, and Conflict, Spring 2023
MATH 6710: Graduate Probability I, Fall 2022
MATH 7710: Topics in Probability: Limits of discrete random structures, Spring 2022
MATH 6710: Graduate Probability I, Fall 2021
MATH 1110: Calculus I, Fall 2021
MATH 6720: Graduate Probability II, Spring 2021
MATH 6710: Graduate Probability I, Fall 2020
MATH 7710: Topics in Probability: Abelian Sandpiles and Abelian Networks, Fall 2020
MATH 4210: Nonlinear Dynamics and Chaos, Spring 2020
MATH 6720: Graduate Probability II, Spring 2020
MATH 6710: Graduate Probability I, Fall 2019
MATH 6720: Graduate Probability II, Spring 2018
MATH 6720: Graduate Probability II, Spring 2017
MATH 1340: Mathematics and Politics, Spring 2016
MATH 4740: Stochastic Processes, Spring 2015
MATH 6710: Probability I, Fall 2014
MATH 4740: Stochastic Processes, Spring 2014
MATH 4740: Stochastic Processes, Spring 2013
MATH 7770: Topics in Probability: Laplacian Growth, Fall 2012
18.312 Algebraic Combinatorics, Spring 2011

Some unpublished papers and notes:

(with Yuval Peres) Internal erosion and the exponent 3/4 describes how an unusual exponent arises from a very simple erosion process in one dimension. The proof we give is due to Kingman and Volkov (2003), who thought of this not as an erosion process but as a model of a gunfight (!)
Orlik-Solomon Algebras of Hyperplane Arrangements, an expository paper proving the basic theorem of Orlik-Solomon and Brieskorn on the cohomology ring of the complement of a complex arrangement, along with some remarks about the associated combinatorics of the intersection lattice.
My Ph.D. thesis, advised by Yuval Peres at Berkeley, used ideas from free boundary problems in PDE to prove limit shapes for both random and deterministic growth models. This topic has advanced a lot in the last fifteen years. Our article Laplacian growth, sandpiles and scaling limits surveys some of the advances.
My senior thesis on the rotor-router model was advised by Jim Propp.
Confounding factors for Hamilton's rule, the final paper for an anthropology class I took in 2002. I found that the rule is surprisingly sensitive to changes in Hamilton's original hypotheses, which casts some doubt on the evolutionary stability of kin selection.
Hall's marriage theorem and Hamiltonian cycles in graphs, the final paper from a spring 2001 reading course with Richard Stanley.
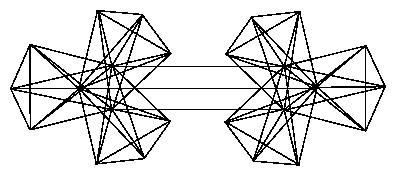
A graph on n=24 vertices having no Hamiltonian cycle, in which every set of k<22 vertices is adjacent to at least k+3 vertices:
Some basic results on Sturmian words, written before I knew that's what they were called. These results are all known in some form. Theorem 1 and the surprising corollary to Theorem 2 go back to Morse and Hedlund (1940).

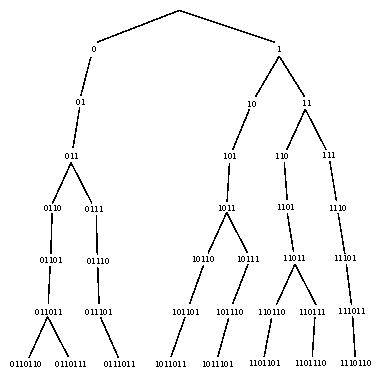
The beginning of the factor tree of the Sturmian word of slope sqrt(2)/2 and intercept zero: