Formally, the dividend yield is equal to the ratio between the annual dividend per share and the price per share. For example, if the price per share is $100, the stock is paying quarterly $1 of dividends per share and the dividend payments are not reinvested in the stock, the annual dividend per share and dividend yield would be equal to $4 and 4%, respectively.

Sometimes secure, stable and low-growth companies distribute a portion of their earnings to some of their share holders because they do not have to reinvest them to sustain growth. These are known as dividends and are usually quoted in terms of the dollar amount each share receives. When trading foreign currency, there exists an interest rate paid per unit of the currency lent or borrowed, analogous to the riskless interest rate, r, considered in the binomial model. This interest rate can be considered as a dividend yield (see tangent). In this lesson we will explore how the previously developed theory changes when the risky asset in the market has dividend payments. For simplicity, the examples presented below are one-step models, however, the results easily extend to the multi-period case.

Suppose the stock price of GM is as in example 3 of the second lesson. But now assume that we know that the company will pay dividend at some point between today and tomorrow (this is a very unrealistic assumption considering the current crisis that "The Big Three" automakers, General Motors, Chrysler and Ford are facing). We consider our time horizon to be 1 day and assume that the dividend payment is 1% of the amount in dollars invested in the stock. Also, as in example 3, we suppose that the riskless interest rate is 2%. The dividend payment between today and tomorrow has an effect on the price of the stock by pushing it up by 1% as shown in the figure below.

By considering this binary tree we see that the rates of return on the stock are now

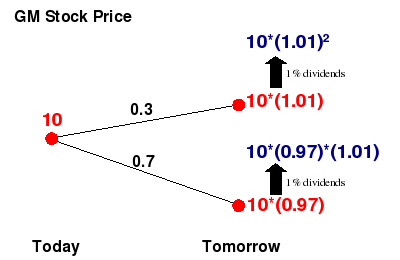
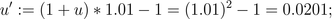
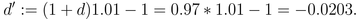
We have then that d'< r=0.02 < u', and according to activity 2 of lesson 3, in this case the market is arbitrage free.

Activity 1

Explain why in this case the strategy: short one unit of the risky asset and invest $10 in the money market account in no longer an arbitrage opportunity. What is the risk neutral probability q^* in this case.

In example 2 we assumed that investors can borrow or lend Euro with no interest rate. Clearly, this assumption is not realistic and it is important to revise this example when the interest rate in Europe is not supposed to be 0. Suppose now that the interest rate in Europe is the same as it is in the US, namely 10%. If this is the case, the arbitrage opportunity described in example 2 is no longer an arbitrage opportunity: if you short one Euro and lend $1.5, tomorrow you will get $1.65 but you will owe 1.1 Euro. If the price of the Euro goes up, once you pay back your debt, you will end up with a net loss of $(1.65*1.1)-$1.65=$0.165. Hence the strategy of "going short on the Euro and long on the Dollar" is not riskless and hence not an arbitrage opportunity anymore. The natural question that arises then is: Is it possible to adjust this strategy to get an arbitrage strategy? or, Is under these circumstances the market arbitrage free?

To answer this question we reason as in the example above. The fact that the interest rate in Europe is 10% is equivalent to saying that the price of one Euro tomorrow is 1.1 times the price today. To account for this fact we can redefine the price and rates of return of the "risky" Euro after one period of time (see the binomial model and figure above):

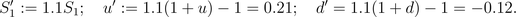
We have then that d'< r=0.1 < u', and according to activity 2 of lesson 3, in this case the market is arbitrage free.

Activity 2

In activity 1 b) of the first lesson, we observed that when the interest rate in the US is 5% and the interest rate in Europe is 0% then the binomial model is arbitrage free. Explain why if the interest in Europe were 31.25% the model would not be arbitrage free and exhibit an arbitrage opportunity in this case.

The general case

In the examples above we assumed that the risky asset pays dividend as a percentage of the value of the asset. Suppose that this percentage is s. As we noticed above we can reduce the problem to the one consider in lesson 1 by adjusting the price of the risky asset and the rates of return, namely

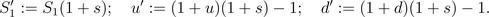
This very simple observation and the first fundamental theorem of asset pricing allow us to conclude that in this case the market is arbitrage-free if and only if d'< r< u'.

In the multi-step case assuming that dividends are paid in every time step and are all equal to s% the the first fundamental theorem of asset pricing as proven in lesson 3, states that d'< r< u' is also a sufficient and necessary condition for the multi-step model to be arbitrage free. However, if dividends are not paid on a regular basis, or are not equal, we can not utilize the binomial model as presented in module 1 for our arguments. To cover this case, we refer the reader to lesson 9 where we study the case when the rates of return of the risky asset are time dependent.