In the previous lesson we observed that by exogenously prohibiting short-selling a complete market may become incomplete. This model was the first example of an incomplete market. In this lesson we will expose an internal characteristic of the market that can make it incomplete which does not involve any constraints on the set of trading strategies. As we mentioned in lesson 8 the models considered so far are one-factor models in the sense that at any time the price variance can be determined by flipping one coin because there are only two possible rates of return. We will see in this section that by allowing more than two rates of return at anytime the market of module 1 might no longer be complete.

Example: General Motors' (GM) stock, multi-factor case

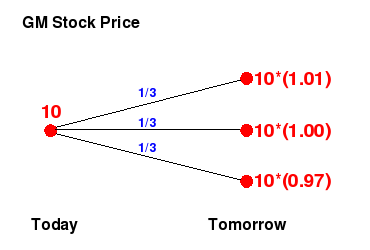
Suppose that GM's stock price behaves as shown in the tree below. On top of each edge in the tree we have placed the corresponding probability. Observe that in this case there are three possible rates of return, either the price goes up by 1%, remains unchanged or goes down by 3%. Each event can occur with the same probability.

Intuitively, the arbitrage-free condition in this case should remain invariant, i.e. d< r< u where, d, r and u are the lowest rate of return, the interest on the dollar and the highest rate of return, respectively. Because if this is not the case, there are arbitrage opportunities like the one presented in example 3 of lesson 2. Suppose for instance that r=0. We argue as we did in the previous lesson. As shown in the figure below, the set of trading strategies with nonpositive initial value and the set of strategies that are nonnegative in the three states of the world can be represented by a semiplane and a cone, respectively.

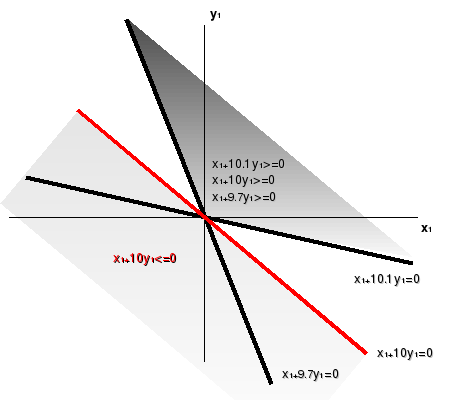
The only strategy in the intersection of the semiplane with the cone is x₁=0=y₁, which is not an arbitrage opportunity. We leave as an exercise for the reader to check that the same holds when assuming r to be any value strictly between d=-0.03 and u=0.01. We might ask then, what is the difference between this multi-factor model and the one factor model considered in the previous lessons? The main difference is that under these hypotheses on the price movement of GM's stock the model is no longer complete. 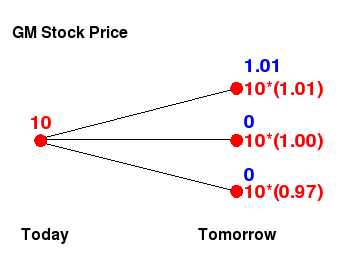To see this consider a call option with maturity of one day and strike price equal to 10. The payoffs under the different states of the world for this option are colored in blue in the tree below.

In order to hedge a position on the call option an investor would like to find an strategy (x₁,y₁) on the money market account and GM stock, respectively. The strategy would solve the following system of three linear equations in two unknowns (see lesson 5).

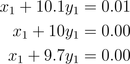
This system has no solution and hence the model is not complete. We can also argue that the model is not complete by using the second fundamental theorem of asset pricing. But before doing so we have to identify what the martingale measures are in this model. A probability measure in this case, can be identified with a triple (p,q,r) that specifies the probability of the price going up, staying constant and going down respectively. Since in our example r=0, the martingale property reduces to having expectation equal to 10, i.e.

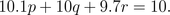
The other conditions on p, q and r come from the restrictions that they have to be probabilities and hence p+q+r=1 and since the original probabilities are all equal to 1/3, in order to have an equivalent probability we must require p,q,r to be strictly positive. It is easy to see that there exist infinitely many triples (p,q,r) that satisfy the conditions above (some examples are p=3/5, q=r=1/5 and p=1/2, q=1/3, r=1/6) and by the second fundamental theorem of asset pricing the model is incomplete. It is important to mention that the fact that there exist at least one triple that satisfies all the conditions guarantees that the model is arbitrage-free by the first fundamental theorem of asset pricing. The same argument can be used to prove that the model is arbitrage-free if and only if -0.03=d< r< u=0.01.

Example: Dollar Vs Euro, Dollar Vs British Pound, non-correlated case

Recall the example presented at the beginning of lesson 8. In that model there were only two possible states of the world either the price of the Euro and pound go up or they go down. This implies that in our model we are assuming that the dollar price of the Euro and the British pound are perfectly correlated. By considering a more general scenario where the interest rates on the Euro, dollar and British pound are all equal to 0, and the correlation hypothesis is dropped we obtain a multi-factor model. In this model we have four states of the world, which we present in the tree below. We have added two branches corresponding to the states of the world in which one price goes up but the other goes down.

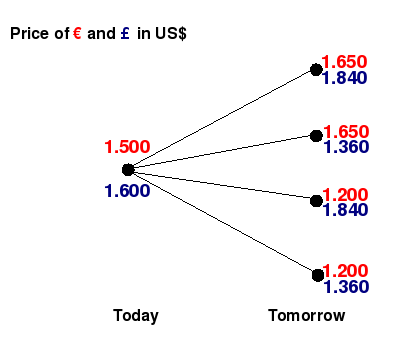
In order to find the risk-neutral measures in this model (see lesson 8), we have to find (p,q,r,s), strictly positive numbers such that

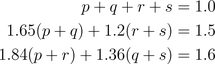
It can be shown that there are infinitely many of such quadruples and hence the market is arbitrage-free but incomplete.

There is a natural extension of the results presented in lessons 7 through 9 to the multi-factor case. We leave this extension as an exercise for the interested reader.